Mechanics: Vectors and Forces in Two-Dimensions
Calculator Pad, Version 2
You are viewing the Legacy Version of The Calculator Pad. We have recently revised and improved The Calculator Pad.
Version 2 is now LIVE! We have more than tripled the number of problems, broken each unit into several smaller, single-topic problem sets, and utilized a random number generator to provide numerical information for each problem. Student answers are automatically evaluated and feedback is instant. And we've maintained the same commitment to providing help via links to existing resources. While the FREE version does all the above, teachers with a Task Tracker subscription can take things a step further. They can modify our pre-made problem sets, write their own problems with our easy-to-use Problem Builder, and use the Calculator Pad to design their own program that expresses their emphasis on the use of mathematics in Physics.
Return to the
Main Page to link into Version 2. Learn more
About Version 2. Or visit the
Store to make a Task Tracker purchase.
Vectors and Forces in 2-D: Problem Set
Problem 1:
For each collection of listed forces, determine the vector sum or the net force.
|
Set A
58 N, right
42 N, left
98 N, up
98 N, down
|
Set B
14 N, left
16 N, up
16 N, down
|
Set C
12 N, up
8 N, down
|
Problem 2:
Hector is walking his dog (Fido) around the neighborhood. Upon arriving at Fidella's house (a friend of Fido's), Fido turns part mule and refuses to continue on the walk. Hector yanks on the chain with a 67.0 N force at an angle of 30.0° above the horizontal. Determine the horizontal and vertical components of the tension force.
Problem 3:
Helen is parasailing. She sits in a seat harness which is attached by a tow rope to a speedboat. The rope makes an angle of 51° with the horizontal and has a tension of 350 N. Determine the horizontal and vertical components of the tension force.
Problem 4:
At one moment during a walk around the block, there are four forces exerted upon Fido - a 10.0 kg dog. The forces are:
Fapp = 67.0 N at 30.0° above the horizontal (rightward and upward)
Fnorm = 64.5 N, up
Ffrict = 27.6 N, left
Fgrav = 98 N, down
Resolve the applied force (Fapp) into horizontal and vertical components, then add the forces up as vectors to determine the net force.
Problem 5:
Jerome and Michael, linebackers for South’s varsity football team, delivered a big hit to the halfback in last weekend’s game. Striking the halfback simultaneously from different directions with the following forces:
FJerome = 1230 N at 53°
FMichael = 1450 at 107°
Determine the resultant force applied by Jerome and Michael to the halfback. (The directions of the two forces are stated as counter-clockwise angles of rotation with East.)
Problem 6:
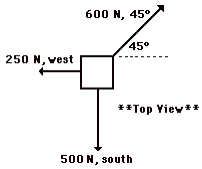
A pack of three Artic wolves are fighting over the carcass of a dead polar bear. A top view of the magnitude and direction of the three forces is shown in the diagram to the right.
a. Determine the resultant or net force acting upon the carcass.
b. Determine the acceleration of the 750-kg polar bear carcass.
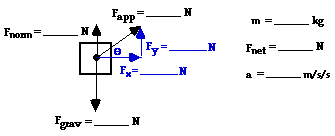
Problem 7:
Lee Mealone is sledding with his friends. Disgruntled by a coarse comment, he decides to separate from the group. He momentarily exerts a 31 N force on the rope which is attached to his 2.5-kg sled. The rope makes an angle of 22° with the nearly frictionless surface. Use the structure provided below to determine the net force on and acceleration of the sled.
Problem 8:
Renee is on Spring Break and pulling her 21-kg suitcase through the airport at a constant speed of 0.47 m/s. She pulls on the strap with 120 N of force at an angle of 38° above the horizontal. Determine the normal force and the total resistance force (friction and air resistance) experienced by the suitcase.
Problem 9:
Ben Laborin works for the South bookstore during the summer months to help prepare for Fall sales. Books from one supplier are shipped to the school in large crates equipped with rope handles on all sides. On one occasion, Ben momentarily pulled with a force of 692 N at an angle of 36.0° above the horizontal to accelerate a 112-kg crate of books. The coefficient of friction between the crates and the vinyl floor is 0.548. Determine the acceleration experienced by the crate. (Suggestion: Begin with a free body diagram.)
Problem 10:
A fire breaks out on a high rise building and a rescue helicopter is called to the scene. The helicopter is equipped with a rescue basket attached by a strong cable to a winch on the helicopter. The rescue basket is lowered to the roof and some occupants of the top floor escape to the roof and enter the basket. As the helicopter takes off, the cable momentarily makes a 78.2° angle to the horizontal, imparting a slight horizontal acceleration to the 621-kg basket and occupants across the roof top. The coefficient of friction between the basket and the roof top is 0.568 and the tension in the cable is 4980 N. Determine the acceleration of the basket and occupants. (Suggestion: Begin with a free body diagram.)
Problem 11:
The custodians clean the field house gym floor between games at the annual Holiday Basketball Classic. Chuck exerts a force on a 1.1-kg push broom as he walks across the floor at a constant speed. The coefficient of friction between the floor and the broom is 0.45 and the broom handle makes an angle of 41° with the horizontal. Determine the amount of force with which Chuck pushes downward (along the handle of the broom) in order to achieve this constant speed motion. (Suggestion: Begin with a free body diagram.)
Problem 12:
Lon Mauer is cleaning up the driveway after mowing the grass. He exerts a sudden downward force of 56.8 N at a constant angle of 54.7° below the horizontal in order to accelerate his 1.05-kg broom from rest. The coefficient of friction between the broom bristles and the driveway is 0.567. Determine the rate of acceleration of the broom. (Suggestion: Begin with a free body diagram.)
Problem 13:
South still displays with great pride the large sign boasting of their 1996 State Championship Girl’s Basketball Team. The 43.1-kg sign hangs from two cables which make an angle of 34.5° with the horizontal. Determine the tension in each of the cables.
Problem 14:
A massive light hangs over the table in Jeremy's dining room. The light is supported by four strong chains which make an angle of 75.0° with the horizontal. The force in each chain is 35.8 N.
a. Determine the force with which a single chain pulls horizontally and vertically upon the light.
b. Determine the mass of the light.
Problem 15:
Troy Ginometri, owner of the local floral shop, displays the sale of the month in the front window of his shop. The 5.6-kg flower pot is suspended by three chains from the ceiling. The chains make an angle of 25° with the vertical. Determine the tension in one of the chains.
Problem 16:
The historic Stanley Center for the Arts in Utica, New York is the proud owner of the world’s largest LED chandelier. The chandelier is 35 feet wide, 17 feet tall and has a mass of 2900 kg. It is directly supported by four cables which make an angle of 63° with the horizontal. Determine the tension in the cables.
Problem 17:

Xavier went rock climbing this past weekend. During one climb through a narrow vertical chimney, he supported his weight by leaning with his back against one wall of the chimney and pushing off the opposite wall with his legs. His left leg made a 26° angle with the horizontal. The coefficient of frcition between his back and the chimney wall is 0.508. Determine the minimum amount of tension which would be required to support the weight of his 86-kg body.
a. Determine the tension in his left leg.
b. Determine the normal force of the chimney wall pushing upon his back.
Problem 18:
Charles Blondin is famous for having crossed the Niagra Falls on an 1100-foot long tight-rope at a height of 160 feet above the gorge. He accomplished the feet several times in a variety of ways – blindfolded, in a sack, carrying his manager on his shoulders, pushing a wheelbarrow and walking on stilts. Suppose the 65-kg Blondin is at the midpoint of the tight-rope and has stopped to cook and eat an omelet. If the tightrope makes an angle of 5° with the horizontal, determine the tension in the rope.
Problem 19:
.gif)
Mom and Dad have tied a rope to Matthew’s sled and are momentarily pulling him through the snow. Dad is pulling with force A and Mom with force B.
A: 54 N at 65° north of east
B: 130 N at 22° south of east
a. Determine the resultant force of A and B.
b. Determine the acceleration that these momentary forces would create for a 39-kg sled and child (assuming negligible friction).
Problem 20:
.gif)
A local automobile dealership has chosen to hang a car outside the entry to their showroom. The car has been modified so that its mass is 645 kg. The car's weight will be supported by a heavy-duty chain which pulls upwards and leftwards. A beam will push outward to balance the wallward pull of the chain. The manufacturer of the chain claims it has a breaking strength of 17400 Newtons. Village code requires that there be a safety factor of 2.20. That is, the actual tension in the chain must be no more than 1/2.20 of the breaking strength. What is the minimum angle (between the chain and the horizontal) at which the dealership can hang the car?
Problem 21:
Lab partners Anna Litical and Noah Formula placed a 0.500-kg glider on their air track and inclined the track at 15.0° above the horizontal. Determine the net force and acceleration of the glider along the frictionless track. Use the structure provided with the free body diagram shown below.
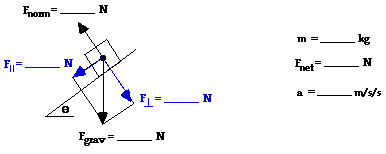
Problem 22:
Anna Litical and Noah Formula now place a 1.38-kg brick on a wooden board and incline the board at 24.0° above the horizontal. The coefficient of friction between the brick and the board is 0. 328. Determine the net force and the acceleration of the brick. Use the structure provided with the free body diagram shown below.
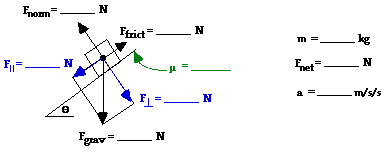
Problem 23:
Ben Laborin exerts a force on an 86-kg crate of books to push it up the ramp of the loading dock at a constant speed of 24 cm/s. The ramp makes an angle of 12° with the horizontal. The coefficient of friction between the crate and the ramp is 0.74. Assuming that Ben is pushing parallel to the inclined plane, determine the force with which he is pushing. (Suggestion: Begin with a free body diagram.)
Problem 24:
The infamous Lombard Street in San Francisco has an abnormally steep block which consists of several tight hairpin turns. On average, the roadway on this block of the street is inclined at 16°. Determine the force which would be required to pull a 23-kg wagon and child up the hill at constant speed. Assume the force is exerted parallel to the road and that friction is negligible.
Problem 25:
In an effort to determine the coefficient of friction between a wood block and a metal track, Anna Litical and Noah Formula place the block on the track and slowly elevate one of its ends until the block finally budges from rest and slides down the incline. Anna and Noah repeat several trials and determine that the block accelerates from its rest position when the track makes an angle of 38° above the horizontal. Use this angle value to determine the coefficient of friction. (Suggestion: Begin with a free body diagram.)
Problem 26:
The Splash Mountain flume ride at Walt Disney World's Magic Kingdom in Orlando, Florida boasts one of the steepest flume rides in the world. The boats plunge 52 feet down a 43-degree incline near the final stage of the ride. Assuming a coefficient of friction of 0.12, determine the acceleration of the boat and riders. (Suggestion: Begin with a free body diagram.)
Problem 27:
Ethan recently found out that the sledding hill on the west side of Lake Bluebird is more of a shredding hill than a sledding hill. He boarded his 60-inch sled and descended the steepest section near the tall trees. Before his ride ended at the location of a tree stump buried a few inches below the snow, he was accelerating at 2.5 m/s/s down the 19° incline. Determine the coefficient of friction between the sled and the snow. (Suggestion: Begin with a free body diagram.)
Return to Overview
View Audio Guided Solution for Problem:
1 |
2 |
3 |
4 |
5 |
6 |
7 |
8 |
9 |
10 |
11 |
12 |
13 |
14 |
15 |
16 |
17 |
18 |
19 |
20 |
21 |
22 |
23 |
24 |
25 |
26 |
27