Mechanics: Vectors and Projectiles
Calculator Pad, Version 2
You are viewing the Legacy Version of The Calculator Pad. We have recently revised and improved The Calculator Pad.
Version 2 is now LIVE! We have more than tripled the number of problems, broken each unit into several smaller, single-topic problem sets, and utilized a random number generator to provide numerical information for each problem. Student answers are automatically evaluated and feedback is instant. And we've maintained the same commitment to providing help via links to existing resources. While the FREE version does all the above, teachers with a Task Tracker subscription can take things a step further. They can modify our pre-made problem sets, write their own problems with our easy-to-use Problem Builder, and use the Calculator Pad to design their own program that expresses their emphasis on the use of mathematics in Physics.
Return to the
Main Page to link into Version 2. Learn more
About Version 2. Or visit the
Store to make a Task Tracker purchase.
Vectors and Projectiles: Problem Set
Problem 1:
Coach Sweeney walks 26 yards to the north along the sideline, pauses, and walks 12 yards back to the south.
a. Determine the distance which Coach moved.
b. Determine Coach's resultant displacement.
Problem 2:
a. Rosa Boat is paddling upstream at 1.25 m/s relative to the water in a river which is flowing at 0.50 m/s relative to the banks of the river. What is the resultant velocity of Rosa's boat (relative to the banks)?
b. Rosa Boat is paddling downstream at 1.25 m/s relative to the water in a river which is flowing at 0.50 m/s relative to the banks of the river. What is the resultant velocity of Rosa's boat (relative to the banks)?
Problem 3:
The takeoff speed of a military aircraft from an aircraft carrier is approximately 170 mi/hr relative to the air. They acquire this speed through a combination of a catapult system present on the aircraft carrier and the aircraft's jet propulsion system. A common strategy is to head the carrier and the plane into the wind. If a plane is taking off from an aircraft carrier which is moving at 40 mi/hr into a 20 mi/hr headwind, then what speed relative to the deck of the aircraft carrier must it obtain to takeoff?
Problem 4:
Claire de Iles is shopping. She walks 16 m to the end of an aisle. She then makes a right hand turn and walks 21 m down the end aisle. Determine the magnitude of Claire's resultant displacement.
Problem 5:
Jim Nazium is walking from lunch to his PE class. He exits the lunchroom and walks 43 m west. He then turns and walks 72 m north down the hallway leading to the locker room. Determine the magnitude and direction of Jim's resultant displacement.
Problem 6:
On her trip from home to school, Karla drives along three streets after exiting the driveway. She drives 1.85 miles south, 2.43 miles east and 0.35 miles north. Determine the magnitude of Karla's resultant displacement.
Problem 7:
Sheila is captain of the Varsity cross country team. During the after-school practice on Tuesday, she led the team on the following run from school to a nearby park where they met the coach for a meeting: 0.68 miles, north; 1.09 miles east; 1.56 miles north; 0.32 miles, west. Determine the magnitude and direction of the team's resultant displacement.
Problem 8:
During the Vector Addition lab, Mac and Tosh start at the classroom door and walk 40.0 m, north, 32.5 m east, 15.5 m south, 68.5 m west, and 2.5 m, north. Determine the magnitude and direction of the resultant displacement of Mac and Tosh.
Problem 9:
Avery, the quarterback of South's Varsity football team, made the most amazing pass in the Homecoming game against cross town rival North. He threw the pass from the exact center of the field to the corner of the end zone, where Jamaal caught it for the game winning score. If the football field is 160 feet wide (sideline to sideline) and it is 60 yards from midfield to the back of the end zone, then how far did the ball travel from Avery's hands to Jamaal's hands.
Problem 10:
Consider the map of the United States below. Given the scale that 1 cm = 340 km, a protractor and a ruler can be used to determine the magnitude and direction for the following trips. All directions are expressed using the counter-clockwise from east convention. For each trip, use the sine, cosine and tangent functions to determine the horizontal and vertical components of the displacement. Be sure to indicate E, W, N, or S as the direction for each component.
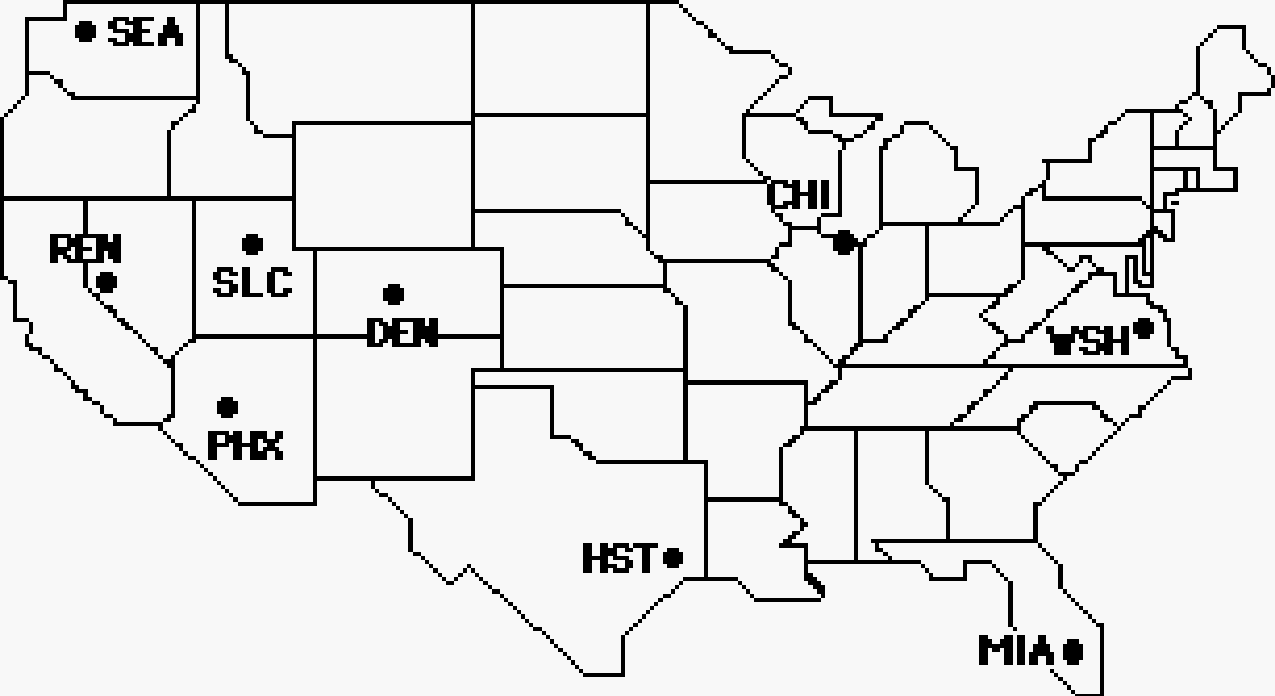
| Trip |
Displacement |
Horiz. Component |
Vert. Component |
| Chicago to Denver |
1430 km, 187° |
|
|
| Reno to Miami |
4030 km, 341° |
|
|
| Seattle to Washington |
3480 km, 344° |
|
|
| Houston to Salt Lake City |
2040 km, 143° |
|
|
Problem 11:
The pilot of a plane flying due north is notified by the flight controller that there is a second plane flying south at about the same altitude and located in the same general area. The pilot is told that the southward bound plane is currently located at a position which is 13.5 km, 102° from her own plane.
a. How many kilometers to the north is the second plane?
b. How many kilometers to the west is the second plane?
c. If the two planes both have an airspeed of 290. km/hr, then how much time will elapse before the planes are side by side?
Problem 12:
A spelunker (person who explores caves) determines that the cave entrance is located 349 m, 253° from her current position. How far south and how far west from her current position is the cave entrance?
Problem 13:
Avery, South's quarterback, throws a pass 36.5 yards at 21° W of S before it is caught by Mitchell with a diving catch. Assuming that the field runs north and south, and that Avery threw the pass from 7.2 yards behind the line of scrimmage, how many yards were gained on the play?
Problem 14:
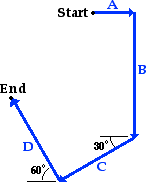
Mia Ander exits the front door of her home and walks along the path shown in the diagram at the right (not to scale). The walk consists of four legs with the following magnitudes:
A = 88 m
B = 272 m
C = 136 m
D = 183 m
Determine the magnitude and direction of Mia's resultant displacement.
Problem 15:
Dora is exploring a cave. She starts at the entrance and makes the following straight line movements:
68 m, south
112 m, 25° north of west (155° CCW)
34 m, south
182 m, 17° south of east (343° CCW)
Determine Dora's position relative to the entrance of the cave. That is, how far and in what direction is Dora from the cave entrance?
Problem 16:
Taylor and Drew finish their last class on the day before Spring break and decide to take a spontaneous road trip. Their trip involves the following movements:
42 miles, 67° north of west (113° CCW)
61 miles, west
23 miles, 17° west of south (253° CCW)
Taylor's car breaks down after the last leg of the trip. How far and in what direction are Taylor and Drew from campus?
Problem 17:
A weather report shows that a tornado was sighted 12 km south and 23 km west of your town. The storm is reported to be moving directly towards your town at a speed of 82 km/hr.
a. What distance from your town was the tornado sighted?
b. Approximately how much time (in minutes and hours) will elapse before the violent storm arrives at your town?
Problem 18:
An airplane begins its journey into Canada from a destination located 285 mi south of the border. The plane flies along a straight-line path at 189 mi/h in a direction of 20.5 degrees west of north. Determine the number of minutes before the plane crosses the border. Assume that the border is aligned directly east and west in the region where the flight takes place.
Problem 19:
Glenda and Harold are attempting to cross a river in a kayak. The river flows due east at 1.9 m/s. Glenda and Harold head the kayak due north and row at 2.4 m/s (relative to the water). The river is 38 m wide at this location.
a. Determine the resultant velocity of the boat - both magnitude and direction.
b. Determine the time for Glenda and Harold to cross the river.
c. How far downstream will the boat be when Glenda and Harold reach the opposite shore?
Problem 20:
Ty Ridlegs boards a paddle boat and heads the boat westward directly across a river. The river flows south at 48 cm/s. Ty paddles the boat with a speed of 98 cm/s.
a. Determine the resultant velocity of the boat - both magnitude and direction.
b. If the river is 22 m wide at this location, then how much time does it take Ty to cross the river? Assume that Ty keeps his paddle boat headed west.
c. How far downstream will Ty be when he reaches the other side of the river?
Problem 21:
Dylan and Sophia are walking along Bluebird Lake on a perfectly calm day. Dylan, determined to impress Sophia by his ability to skip rocks, picks up the flattest rock he can find and gives it a sidearm launch from the edge of the water. The rock acquires a completely horizontal velocity of 26 m/s from a height of 0.45 m above the water surface.
a. How much time does it take the rock to fall to the water surface?
b. How far from the edge of the water does the rock travel before it makes its first skip?
Problem 22:
In an effort to create a cannonball-style splash, eight-year old Matthew runs off the edge of the board of the high dive at 4.6 m/s and falls 2.3 m to the water below.
a. Determine the time for Matthew to fall the 2.3 m to the water.
b. What horizontal distance from the edge of the board will Matthew plunge into the water?
c. With what speed does Matthew enter the water?
Problem 23:
Ima Peode wishes to throw a 2.8-kg pumpkin horizontally off the top of the school roof in order to hit Mr. H's car. The car is parked a distance of 13.4 m away from the base of the building below the point where Ima is standing. The building's roof is 10.4 m high. Assuming no air resistance, with what horizontal speed must Ima toss the pumpkin in order to hit Mr. H's car.
Problem 24:
The La Quebrada Cliff Divers provide daily entertainment for the crowds at Acapulco, Mexico. As a group of professional high divers, they dive off the cliff of La Quebrada and fall 45.1 m (148 feet) to the water below. More than an act of bravery, the cliff divers must time their dive so that they hit the water when the crest of an incoming wave has arrived. Determine the speed with which Pedro must run off the cliff in order to land in the water a horizontal distance of 17.8 m from the edge of the cliff.
Problem 25:
An emergency relief plane is dropping a care package from a plane to a group of medical personnel working for a relief agency in an African village. The package is designed to land in a small lake, inflate an attached raft upon impact, and finally resurface with the raft side down. The plane will be moving horizontally with a ground speed of 59.1 m/s. The package will be dropped a horizontal distance of 521 m from the intended target location. At what altitude above the pond must the plane be flying in order to successfully accomplish this feat?
Problem 26:
The Choo Choo Restaurant in DesPlaines, IL is a 50’s style diner which is notorious for the delivery of food from the kitchen to the dining room by an O-scale model train. Dinner baskets filled with hot dogs, hamburgers, French fries and the like are mounted to the tops of flatbed train cars and transported to table tops. On Matthew’s fifth birthday, a French fry rolled off the top of the pile on a tight turn moving at a speed of 1.25 m/s and fell to the floor.
a. Determine the time for the French fry to fall 113 cm from the top of the pile to the floor.
b. Determine the horizontal displacement of the fry from the edge of the track.
c. Determine the speed of the French fry upon striking the floor.
Problem 27:
Aaron Agin and Bud Derfenger are lab partners who last year earned a reputation for breaking beakers, spilling acid, mixing the wrong chemicals, breaking thermometers and accidentally lighting Sophia’s hair on fire with a Bunsen burner. And now to the delight of the physics class, Mr. H has made the mistake of allowing them to partner again. In a recent lab which utilized expensive tracks and carts, Aaron and Bud lived up to their reputation. Despite strong warnings from Mr. H, they allowed a cart to roll off the track and then off the table with a speed of 208 cm/s. The crash of the cart to the floor a horizontal distance of 96.3 cm from the table’s edge turned the entire classroom silent. Use this information to determine the height of the lab tables in Mr. H’s lab.
Problem 28:
Sharon Steady and Al Wayskachon won South’s recent egg toss contest held during Homecoming week. In their winning toss, Sharon gave the egg an underhand toss, releasing it with a velocity of 8.06 m/s at an angle of 30° to the horizontal. To the pleasure of the crowd, Al caught the egg at the same height as the toss without even a fracture to its shell.
a. Calculate the horizontal and vertical components of the initial velocity.
b. Calculate the time for the egg to reach the midpoint of the trajectory.
c. Calculate the total time the egg is in the air.
d. Calculate the horizontal distance which the egg traveled from Sharon to Al.
e. Calculate the height of the egg (relative to the releast point) when it was at the peak of its trajectory.
Problem 29:
Li Ping Phar, the famous Chinese ski jumper, leaves the ramp with an initial velocity of 34.9 m/s at an angle of 35°.
a. Determine the total time of flight.
b. Determine the horizontal displacement.
c. Determine the peak height (relative to the starting height). Assume that Li lands at the same height as the top of the ramp and that Li is a projectile.
Problem 30:
A tennis player stretches out to reach a ball that is just barely above the ground and successfully 'lobs' it over her opponent's head. The ball is hit with a speed of 18.7 m/s at an angle of 65.1 degrees.
a. Determine the time that the ball is in the air.
b. Determine the maximum height which the ball reaches.
c. Determine the distance the ball travels horizontally before landing.
Problem 31:
On New Year’s eve of 2007, Robbie Maddison set the world record for the longest motorcycle jump, traveling 98.3 m through the air from ramp to ramp. (The record has since been broken several times by Maddision himself.) Assuming a launch angle of 45°, insignificant air resistance and a landing location at the same height as the launch height, determine the speed with which Maddison left the ramp.
Problem 32:
Mr. Udadi takes his three children to the park for some summertime recreation. Olive Udadi is enjoying swinging and jumping. On one jump, Olive leaves the swing at a 30° angle to the horizontal with a speed of 2.2 m/s. She lands on the ground a horizontal distance of 1.09 m from the launch location.
a. Determine the horizontal and the vertical components of the initial velocity.
b. Determine the time which Olive is in the air.
c. Determine the vertical height (relative to the landing location) from which Olive jumps from the swing.
Problem 33:
In an apparent effort to earn an appearance on the Destroyed in Seconds show, Caleb attempts a bicycle maneuver in which he jumps between two ramps whose elevated edges are located a distance of 1.8 meters apart. The ramps are angled at 35° and located at the same height. Determine the speed (in m/s and mi/hr) that Caleb must acquire to accomplish this stunt. (Given: 1.00 m/s = 2.24 mi/hr)
Problem 34:
Albert is South’s star punter for the varsity football team. His best hang time this past season was for a punt which he kicked at 74° above the horizontal. The punt had a 6.2 second hang time.
a. Determine the speed at which the ball was punted.
b. Determine the horizontal distance which the ball traveled.
Return to
Overview
View Audio Guided Solution for Problem:
1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | 25 | 26 | 27 | 28 | 29 | 30 | 31 | 32 | 33 | 34